At SmartCapitalMind, we're committed to delivering accurate, trustworthy information. Our expert-authored content is rigorously fact-checked and sourced from credible authorities. Discover how we uphold the highest standards in providing you with reliable knowledge.
What is a Trimmed Mean?
A trimmed mean is a strategy that is sometimes used in creating useful averages. This particular approach normally involves disregarding the most extreme values associated with a given calculation before attempting to determine the mean figure for that situation. By essentially discarding the highest and lowest values, it is often thought that the resulting trimmed mean is actually more accurate and useful in terms of making decisions regarding financial investments or business deals.
The actual process of determining a trimmed mean requires that all relevant data be identified, arranging that data in a sequence that begins with the smallest or lowest value and advances to the highest or largest applicable value. A simple example would be tabulating the scores rendered by judges during some type of competition. Assuming there are five judges that use a ten point scale to score performance levels, arriving at the trimmed mean would mean disregarding the lowest and highest scores, and focusing attention on the three remaining scores.
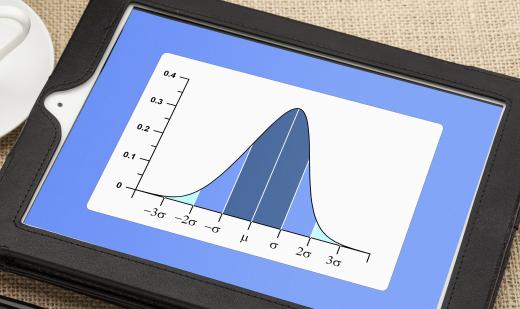
Assuming that the judges involved submitted scores of 4, 6, 7, 8, and 9 for a particular performance, identifying the trimmed mean would mean dropping the scores of 4 and 9 from consideration. From that point, this would mean averaging the scores of 6, 7, and 8 to arrive at a trimmed mean of 7. In doing so, the process helps to minimize any potential of bias for or against the competitor and arrives at a mean score that is considered to be more balanced and likely a better representation of that individual’s performance.
The same general approach can be utilized when evaluating the performance of a given investment. By identifying the security price that is applied over a fixed number of successive periods, it is possible to disregard the highest and lowest of those prices, then average the remaining prices to arrive at the mean price for the entire time frame under consideration. By not including the lowest and highest prices in the calculation, the investor is able to disregard unusual circumstances that caused the stock price to either plummet or rise in a manner that is out of step with the normal performance range of that security. By arriving at the trimmed mean price, the investor has a better idea of how that security is likely to perform under typical market conditions and can decide whether to buy, hold, or sell that security as a result of the findings and hopefully move closer to achieving his or her financial goals.
AS FEATURED ON:
AS FEATURED ON:









Discuss this Article
Post your comments